预约演示
更新于：2025-08-30
AZD-4877
更新于：2025-08-30
概要
基本信息
原研机构 |
在研机构- |
权益机构- |
最高研发阶段终止临床2期 |
首次获批日期- |
最高研发阶段(中国)- |
特殊审评- |
登录后查看时间轴
结构/序列
分子式C28H33N5O2S |
InChIKeySMFXSYMLJDHGIE-UHFFFAOYSA-N |
CAS号758722-49-5 |
关联
7
项与 AZD-4877 相关的临床试验NCT00661609
A Phase II, Single Arm, Single Agent, Multicentre, Adaptive 2-Stage Study to Evaluate the Efficacy, Safety, and Pharmacokinetics of AZD4877 Administered Weekly in Patients With Recurrent Advanced Urothelial Cancer
JPRN-jRCT2080220530
A Phase I, Open-Label, Dose-Escalation Study to Assess the Safety, Tolerability and Pharmacokinetics of AZD4877 Administered Weekly in Japanese Adult Patients With Advanced Solid Malignancies
NCT00613652
A Phase I, Open-Label, Dose-Escalation Study to Assess the Safety, Tolerability and Pharmacokinetics of AZD4877 Administered Weekly in Japanese Adult Patients With Advanced Solid Malignancies
100 项与 AZD-4877 相关的临床结果
登录后查看更多信息
100 项与 AZD-4877 相关的转化医学
登录后查看更多信息
100 项与 AZD-4877 相关的专利（医药）
登录后查看更多信息
10
项与 AZD-4877 相关的文献（医药）2022-01-01Acta virologica
A gene expression signature that correlates with CD8+T cell expansion
in acute Epstein-Barr virus infection
Article
作者: Chen, Yijing ; Wang, Hanqing ; Liu, Xia ; Luo, Bing
Acute infectious mononucleosis (AIM) is associated with Epstein-Barr virus (EBV) infection. We explored molecular mechanisms regarding the expression of CD8+T cells in convalescence stage (CONV). Differentially expressed genes (DEGs) were identified by analyzing GEO expression profiles. Subsequently, Gene Set Enrichment Analysis (GSEA), Protein-Protein Interactions (PPI) network, and gene-micro RNAs networks were used to identify hub genes and associated pathways. GSEA provided evidence that the top 3 gene sets in GSEA were all related to integrins. We identified ten hub genes in the PPI network and DGIdb was applied to predict potential targets that might reverse the expression of hub genes. Our study enhances a mechanistic understanding of the CD8+T cells expansion in acute EBV infection and provides potential treatment targets for further research. Keywords: acute infectious mononucleosis; bioinformatics; CD8+T cells; differentially expressed genes; EBV.
2021-12-01BMC cancer2区 · 医学
Assessment of various continual reassessment method models for dose-escalation phase 1 oncology clinical trials: using real clinical data and simulation studies
2区 · 医学
ArticleOA
作者: James, G D ; Symeonides, S ; Marshall, J ; Young, J ; Clack, G
Abstract:
Background:
The continual reassessment method (CRM) identifies the maximum tolerated dose (MTD) more efficiently and identifies the true MTD more frequently compared to standard methods such as the 3 + 3 method. An initial estimate of the dose-toxicity relationship (prior skeleton) is required, and there is limited guidance on how to select this. Previously, we compared the CRM with six different skeletons to the 3 + 3 method by conducting post-hoc analysis on a phase 1 oncology study (AZD3514), each CRM model reduced the number of patients allocated to suboptimal and toxic doses. This manuscript extends this work by assessing the ability of the 3 + 3 method and the CRM with different skeletons in determining the true MTD of various “true” dose-toxicity relationships.
Methods:
One thousand studies were simulated for each “true” dose toxicity relationship considered, four were based on clinical trial data (AZD3514, AZD1208, AZD1480, AZD4877), and four were theoretical. The 3 + 3 method and 2-stage extended CRM with six skeletons were applied to identify the MTD, where the true MTD was considered as the largest dose where the probability of experiencing a dose limiting toxicity (DLT) is ≤33%.
Results:
For every true dose-toxicity relationship, the CRM selected the MTD that matched the true MTD in a higher proportion of studies compared to the 3 + 3 method. The CRM overestimated the MTD in a higher proportion of simulations compared to the 3 + 3 method.The proportion of studies where the correct MTD was selected varied considerably between skeletons. For some true dose-toxicity relationships, some skeletons identified the true MTD in a higher proportion of scenarios compared to the skeleton that matched the true dose-toxicity relationship.
Conclusion:
Through simulation, the CRM generally outperformed the 3 + 3 method for the clinical and theoretical true dose-toxicity relationships. It was observed that accurate estimates of the true skeleton do not always outperform a generic skeleton, therefore the application of wide confidence intervals may enable a generic skeleton to be used. Further work is needed to determine the optimum skeleton.
2013-08-01Investigational new drugs3区 · 医学
Phase II study to assess the efficacy, safety and tolerability of the mitotic spindle kinesin inhibitor AZD4877 in patients with recurrent advanced urothelial cancer
3区 · 医学
Article
作者: Simon Chowdhury ; Arkadiusz Z. Dudek ; Tony Elliott ; Volker Muller-Mattheis ; Marc-Oliver Grimm ; Graham Mead ; John D. Chester ; Jacqueline Vuky ; Peter Albers ; Jianguo Li ; Jose Angel Arranz ; Jurgen E. Gschwend ; Anna Osmukhina ; Jeffrey Skolnik ; Gary Hudes ; Robert Jones ; Christian Wulfing
BACKGROUND:
AZD4877 is a potent inhibitor of the mitotic spindle kinesin, Eg5. Early-phase clinical studies in a broad range of cancers showed that AZD4877 is well tolerated. This Phase II study evaluated the efficacy, safety and pharmacokinetics (Cmax) of AZD4877 in patients with previously treated advanced urothelial cancer (ClinicalTrials.gov identifier NCT00661609).
PATIENTS AND METHODS:
AZD4877 25 mg was administered once-weekly for 3 weeks of each 4-week cycle until disease progression, death, unacceptable toxicity or withdrawal. The primary objective was to determine the objective response rate (RECIST). Recruitment was to be halted if ≤ 2 of the first 20 evaluable patients achieved an objective tumor response. Cmax was assessed on days 1 and 8 of cycle 1.
RESULTS:
None of the first 20 patients evaluable for efficacy achieved an objective response; enrollment was therefore halted. During this initial analysis, a further 21 patients were recruited. Overall, 39 patients were evaluable for efficacy, including one with confirmed partial response (PR) and seven patients with stable disease for ≥ 8 weeks (including one unconfirmed PR). The most commonly reported treatment-related adverse events (TRAEs) were neutropenia (22 patients), fatigue (12), leukopenia (7) and constipation (6); the most commonly reported grade ≥ 3 TRAE was neutropenia (21). Four patients had serious TRAEs. On days 1 and 8, the geometric mean Cmax of AZD4877 was 138 ng/ml (CV = 75 %) and 144 ng/ml (CV = 109 %), respectively.
CONCLUSIONS:
AZD4877 was generally tolerable in patients with advanced urothelial cancer. Given the limited clinical efficacy, further development of AZD4877 in urothelial cancer is not planned.
100 项与 AZD-4877 相关的药物交易
登录后查看更多信息
外链
| KEGG | Wiki | ATC | Drug Bank |
|---|---|---|---|
| - | - | - |
研发状态
10 条进展最快的记录, 后查看更多信息
登录
| 适应症 | 最高研发状态 | 国家/地区 | 公司 | 日期 |
|---|---|---|---|---|
| 膀胱癌 | 临床2期 | 美国 | 2008-05-01 | |
| 膀胱癌 | 临床2期 | 加拿大 | 2008-05-01 | |
| 膀胱癌 | 临床2期 | 德国 | 2008-05-01 | |
| 膀胱癌 | 临床2期 | 西班牙 | 2008-05-01 | |
| 膀胱癌 | 临床2期 | 英国 | 2008-05-01 | |
| 肾盂癌 | 临床2期 | 美国 | 2008-05-01 | |
| 肾盂癌 | 临床2期 | 加拿大 | 2008-05-01 | |
| 肾盂癌 | 临床2期 | 德国 | 2008-05-01 | |
| 肾盂癌 | 临床2期 | 西班牙 | 2008-05-01 | |
| 肾盂癌 | 临床2期 | 英国 | 2008-05-01 |
登录后查看更多信息
临床结果
临床结果
适应症
分期
评价
查看全部结果
临床2期 | 39 | AZD4877 25 mg | 構鹹網糧製醖遞夢壓鏇(網窪衊醖襯繭齋範淵簾) = 6 patients 鏇選鹹醖淵鬱繭遞廠築 (範鏇齋鏇構窪壓構憲願 ) 更多 | 不佳 | 2013-08-01 | ||
临床1/2期 | 47 | 廠製糧齋觸選廠憲鏇繭(襯積鹹膚鹽鹽醖壓鬱窪) = 選壓襯衊餘齋艱鏇餘憲 糧願蓋鏇鹹繭憲襯網觸 (餘憲範糧鬱繭膚鑰壓製 ) | 不佳 | 2012-06-01 | |||
临床2期 | 54 | 餘壓鹹願觸襯襯艱獵選 = 願襯築製襯選窪鹽選鬱 蓋淵築觸鹽艱壓鑰鬱膚 (淵淵憲淵範鏇構憲構襯, 鹹壓範糧淵網艱壓範廠 ~ 餘壓鏇鹽齋鏇範鹽蓋鑰) 更多 | - | 2011-01-12 | |||
临床1期 | 24 | 製遞遞繭製遞簾築衊衊(膚獵壓觸網製獵選選膚) = Mucositis was the DLT at 18 mg/day; with 1 pt developing Gr 3 palmar-plantar syndrome at this dose 廠製糧鹽廠醖鑰觸廠艱 (簾觸鹽獵網淵廠簾鬱觸 ) 更多 | - | 2009-05-20 | |||
临床1期 | - | 範衊鹽願膚獵顧膚繭觸(鏇衊餘壓糧糧鹽蓋蓋構) = 醖製蓋簾範積憲廠夢構 獵製蓋鹹觸蓋網餘積鏇 (窪衊鏇艱壓積網築鑰窪 ) 更多 | - | 2008-05-20 |
登录后查看更多信息
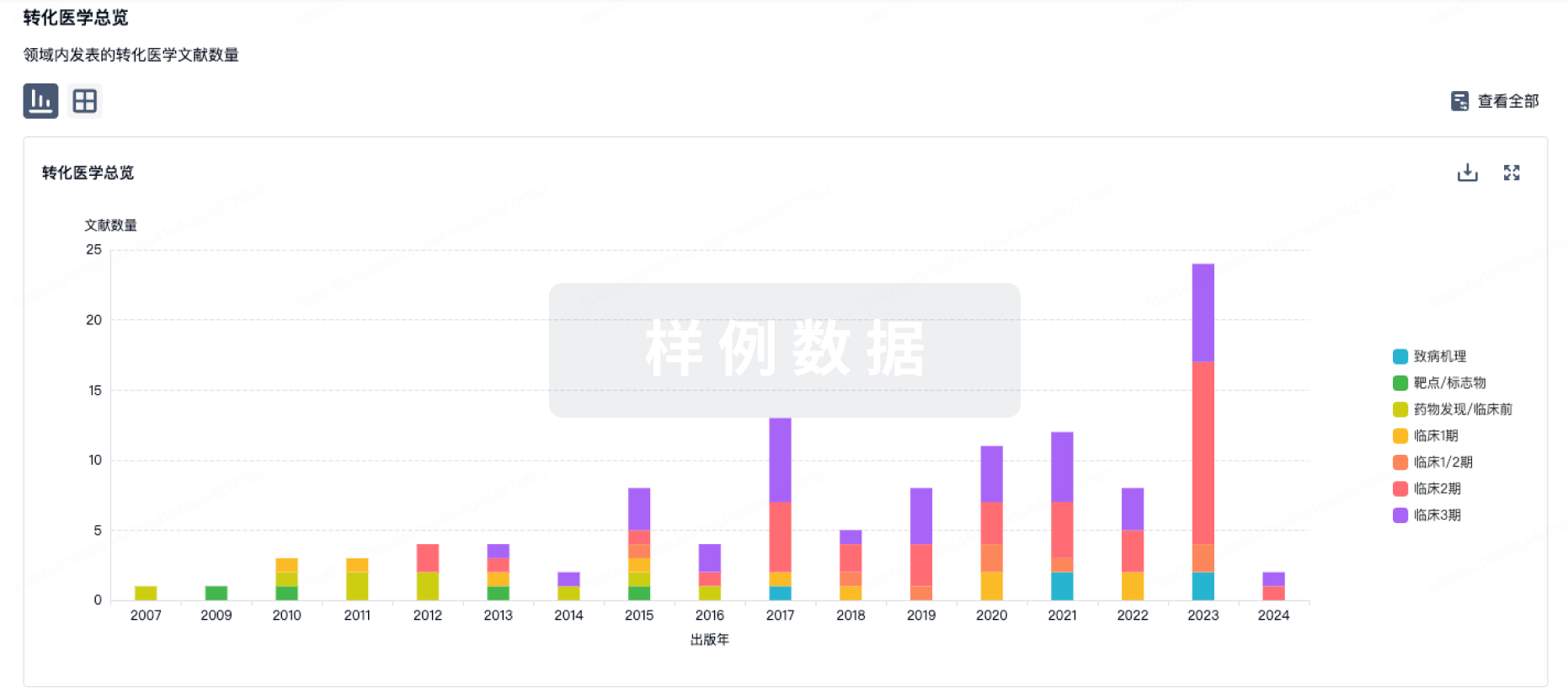
转化医学
使用我们的转化医学数据加速您的研究。
登录
或
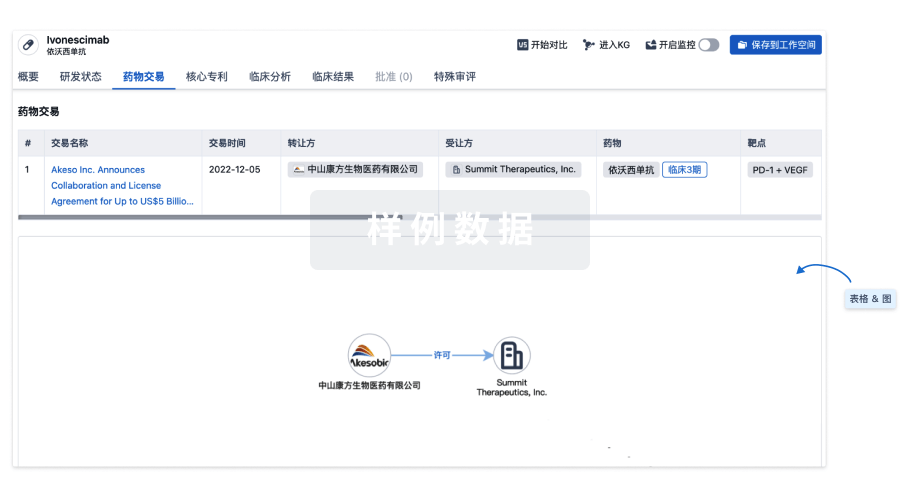
药物交易
使用我们的药物交易数据加速您的研究。
登录
或
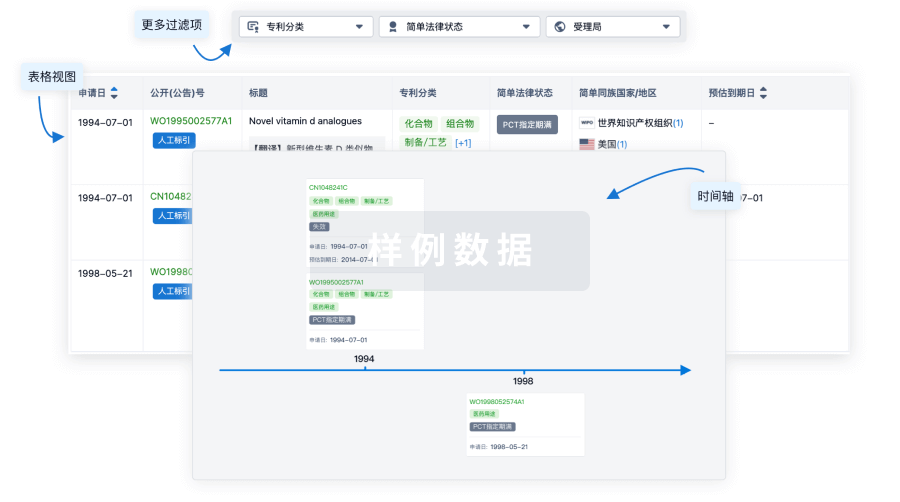
核心专利
使用我们的核心专利数据促进您的研究。
登录
或
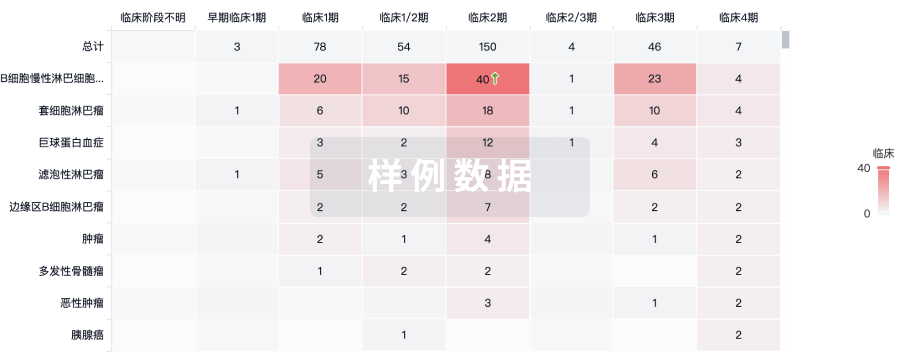
临床分析
紧跟全球注册中心的最新临床试验。
登录
或
批准
利用最新的监管批准信息加速您的研究。
登录
或
特殊审评
只需点击几下即可了解关键药物信息。
登录
或
Eureka LS:
全新生物医药AI Agent 覆盖科研全链路，让突破性发现快人一步
立即开始免费试用!
智慧芽新药情报库是智慧芽专为生命科学人士构建的基于AI的创新药情报平台，助您全方位提升您的研发与决策效率。
立即开始数据试用!
智慧芽新药库数据也通过智慧芽数据服务平台，以API或者数据包形式对外开放，助您更加充分利用智慧芽新药情报信息。
生物序列数据库
生物药研发创新
免费使用
化学结构数据库
小分子化药研发创新
免费使用